| PHY 252 |
Dynamics of Elementary Particles
|
Introduction
Elementary particles are everywhere around us. Apart
from the standard matter particles proton, neutron and electron, hundreds of
other particles have been found, produced in cosmic ray interactions
in the atmosphere or by accelerators. Hundreds of charged particles traverse
our bodies per second, and some will damage our DNA, one of the reasons
for the existence of a sophisticated DNA repair mechanism in the cell.
The data for this experiment is in the form of a bubble
chamber photograph which
shows bubble tracks made by elementary particles as they traverse liquid
hydrogen. In the experiment under study, a beam of low-energy
negative pions (p- beam) hits a
hydrogen (p for proton) target in the form of a container with
liquid hydrogen normally kept just below its boiling point (T=20 K).
As the pions enter the detector a piston slightly decompresses the liquid so
it becomes ``super-critical'' and starts boiling (i.e. bubble formation) first
at the ionization (protons and electrons)
trails left by the charged particles traversing the liquid.
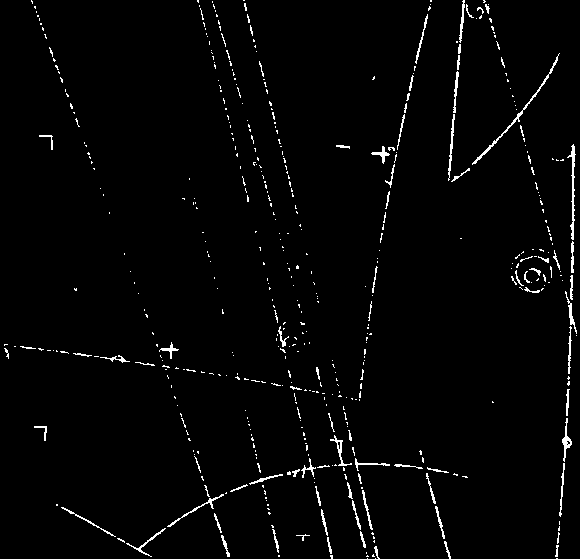
Figure 1: Photograph of the interaction between a
high-energy p--meson from the Berkeley Bevatron
accelerator and a proton in a liquid hydrogen bubble chamber, which produces two
neutral particles L0 and K0,
which are short-lived and decay into charged particles a bit
further. |
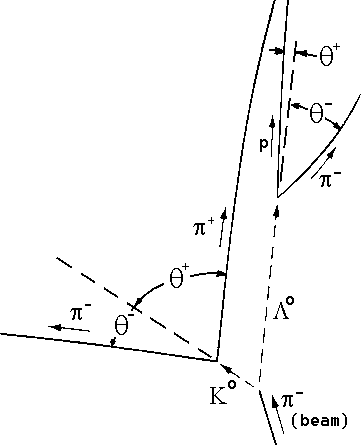
Figure 2: illustration of the interaction, and identification of
bubble trails and variables to be measured in the
photograph in Figure 3.
|
The reaction photographed in Figure 1
shows the production of a pair of neutral particles (that do not
leave a ionized trail in their wake), which after a short while decay into
pairs of charged particles:
p- + p
� L0 +
K0 ,
where the neutrals decay as follows:
L0 �
p + p- ,
K0 �
p+ + p- .
In this experiment, we know the masses of the proton
(mp = 938.3 MeV/c2)
and the pions (mp+ =
mp- =
139.4 MeV/c2) precisely, and
will determine the masses of the L0 and the
K0, also in these mass energy units.
Momentum measurement
In order to ``reconstruct'' the interaction completely, one uses the conservation
laws of (relativistic) momentum and energy, plus the knowledge of the initial
pion beam parameters (mass and momentum). In order to measure momenta of the
produced charged particles, the bubble chamber is located inside a magnet that
bends the charged particles in helical paths. The 1.5 T magnetic field is
directed up out of the photograph. The momentum p of each particle is directly
proportional to the radius of curvature r, which in turn can be calculated
from a measurement of the ``chord length'' l and sagitta s as:
r = [l2/(8s)] + [s/2] .
Note, that the above is strictly true only if all momenta are perfectly in the plane
of the photograph; in actual experiments stereo photographs of the interaction are
taken to be able to reconstruct the interaction in all three dimensions. The
interaction in this photograph was specially selected for its planarity.
In the reproduced photograph the actual radius of curvature R of the track
in the bubble chamber is multiplied by the magnification factor m,
r = mR.
For the reproduction in Figure 3 m = height of photograph (in mm)
divided by 173 mm.
The momentum p of the particles is proportional to their radius of curvature
R in the chamber. To derive this relationship for relativistic particles we
begin with Newton's law in the form:
F =
dp/dt = e v×B
(Lorentz' force).
Here the momentum (p) is the relativistic momentum
mvg, where the relativistic
g-factor is defined in the usual way
g = [�(1-v2/c2)]-1.
Thus, because the speed v is constant:
F = dp/dt =
d(mvg)/dt =
mg dv/dt =
mg (v2/R)(-r) =
e v B (-r) ,
where r is the unit vector in the
radial direction.
Division by v on both sides of the last equality finally yields:
mgv / R =
p / R = e B ,
identical to the non-relativistic result! In atomic units we
find:
|
p c (in eV) = c R B ,
thus
p (in MeV/c) = 2.998×108
R B ×10-6 =
300 R(in m) B(in T) |
(1) |
Measurement of angles
Draw straight lines from the point of primary interaction to
the points where the L0 and the
K0 decay. Extend the lines beyond the decay vertices.
Draw tangents to the four decay product tracks at the two vertices. (Take care
drawing these tangents, as doing it carelessly is a source of large errors.) Use
a protractor to measure the angles of the decay product tracks relative to the
parent directions (use Fig. 3 for measurements and Fig. 2 for definitions).
Analysis
The laws of relativistic dynamics relevant to this calculation are written
below. We use the subscripts zero, plus, and minus to refer to the charges of
the decaying particles and the decay products.
|
p+sinq+ =
p-sinq- |
(2) |
|
p0 =
p+cosq+ +
p-cosq- |
(3) |
|
E0 = E+ + E-,
where
E+ =
�(p+2c2 +
m+2c4) ,
and
E- =
�(p-2c2 +
m-2c4) | |
|
m0c2 =
�(E02 -
p02c2) | |
Note that there is a redundancy here. That is, if
p+, p-,
q+, and
q- are all known, equation (2) is not
needed to find m0. In our two-dimensional case we have two
equations (2 and 3), and only one unknown quantity
m0, and the system is over-determined. This is
fortunate, because sometimes (as here) one of the four measured quantities
will have a large experimental error. When this is the case, it is usually
advantageous to use only three of the variables and to use equation (2)
to calculate the fourth. Alternatively, one may use the
over-determination to ``fit'' the m0, and to determine it
more precisely.
- Focus your attention on the K0 decay. Measure three of the quantities
r+, r-, q+,
and q-. Omit the one which you believe
would introduce the largest experimental error if used to determine mK.
- Use the magnification factor m to calculate the actual radii
R and equation (1)
to calculate the momenta (in MeV/c) of one or both pions.
- Use the equations above to determine the rest mass (in MeV/c2) of the
K0.
- Carefully estimate the error in your result from the errors in the
measured quantities.
- Now turn your attention to the L0: the
proton track is too straight to be well measured in curvature. Also,
q+ is small, and the
value of mL is quite sensitive to
this measurement. Assume that q+ =
(0.32±0.05)� (check this with your
protractor). Measure r- and q-.
- Calculate mL and its error the
same way as for the K0.
- Finally, compare your values with the accepted mass values
(the world average), and discuss.
Bonus - 25% extra credit
Calculate the momenta for both neutral particles, and hence find their
lifetimes, both in the laboratory, and in their own rest-frames. Compare the
latter with the accepted values.
Figure 3 (Next Page): Photograph of the interaction between a
high-energy p--meson from the Berkeley
Bevatron accelerator and a proton in a liquid hydrogen bubble
chamber. The interaction produces two neutral particles
L0 and K0,
which are short-lived and decay into charged particles a bit
further. The photo covers an area (H×W) of 173 mm ×
138 mm of the bubble chamber. In this enlargement m =
actual height (in mm) of the photograph /173 mm.
Revised: August 14, 1998



